The GBS code

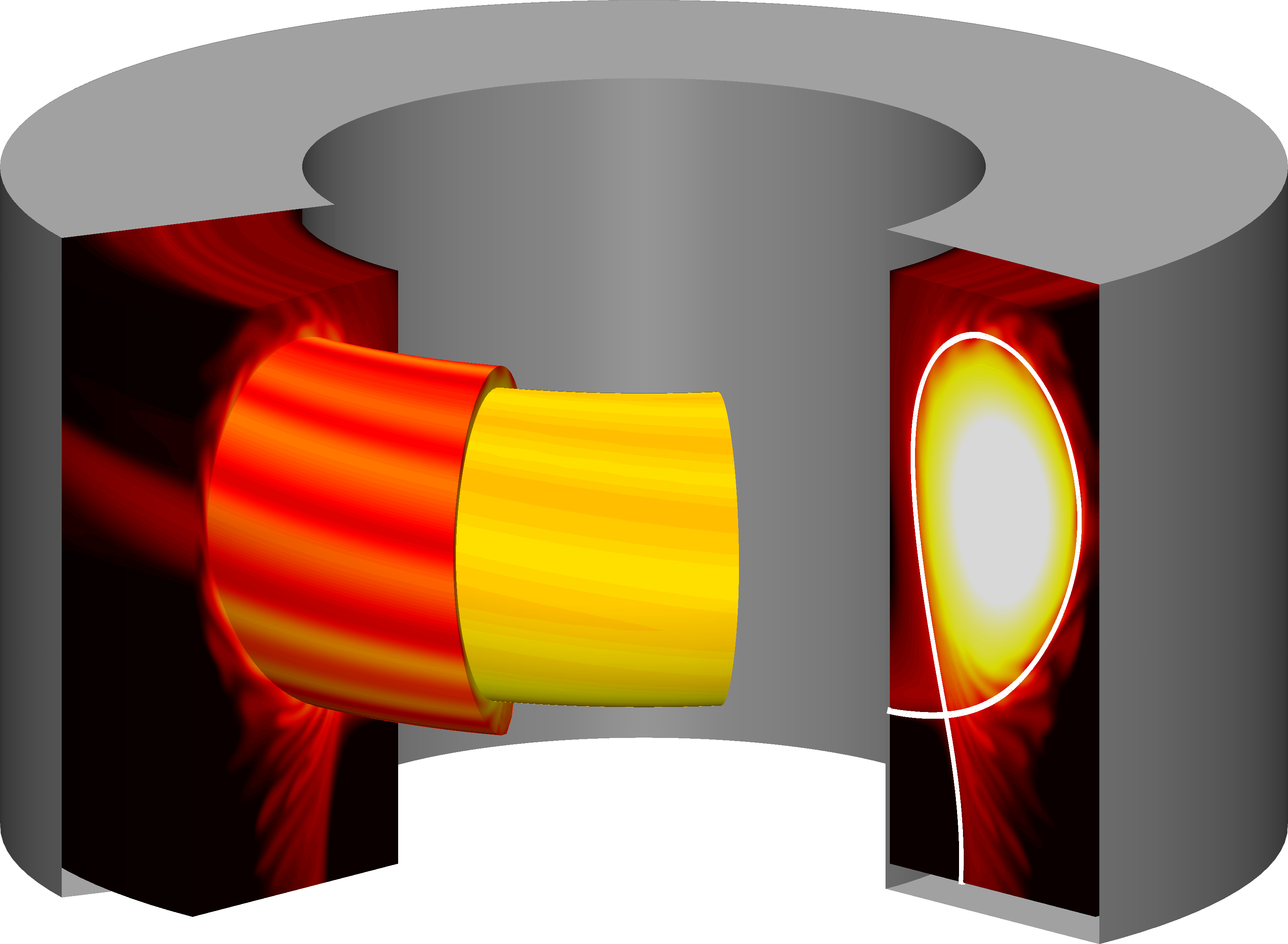
GBS is a first-principles, three-dimensions, flux-driven, global, turbulent code that evolves the drift-reduced Braginskii equations. The GBS code has been used in the past years to simulate plasma turbulence in the boundary of tokamak devices in both limited as well as single-null, double-null and snowflake diverted geometries.

GBS uses a fourth order Runge-Kutta scheme for the time evolution. All plasma quantities are evaluated on a uniform Cartesian grid using cylindrical coordinates. All spatial operators are discretized using a fourth order finite differences scheme except for the Poisson bracket that uses an Arakawa scheme. To avoid typical checkerboard patterns, the code uses two staggered grids to evolve on one hand the density, ion and electron temperature, vorticity and electrostatic potential and on the other hand, the ion and electron velocities and the magnetic potential.

The code is currently parallelized with a 3D domain decomposition using MPI. Current developments are made to port it to GPUs.

Representation of the plasma electron pressure from a GBS simulation of the TCV tokamak.

Attention

The main references for the GBS code are:

• M. Giacomin, et al., The GBS code for the self-consistent simulation of plasma turbulence and kinetic neutral dynamics in the tokamak boundary, Journal of Computational Physics, 464, 111294 (2022)
• P. Ricci et al., Simulation of Turbulence in scrape-off layer conditions: the GBS code, simulation results and code validation, Plasma Physics and Controlled Fusion, 54, 124047 (2012)

We ask you to cite them in any publication/presentation in which you present scientific results that used the GBS code.